CBSE Class 10th CBSE Class 10th Relationship between Zeroes and Coefficients of a Polynomial Details & Preparations Downloads
In the enchanting realm of Class 10 mathematics, the study of polynomials unveils a captivating connection between their zeroes and coefficients. This intricate relationship not only deepens our understanding of algebra but also provides valuable insights into the behavior of these mathematical expressions. Let's embark on a journey to explore the nuanced relationship between the zeroes and coefficients of a polynomial.
Harmony in Equations: Unveiling the Class 10 Enigma - Exploring the Dynamic Relationship between Polynomial Zeroes and Coefficients
Relationship Between the Zeros and Coefficients of a Polynomial
A real number say “a” is a zero of a polynomial P(x) if P(a) = 0. The zero of a polynomial is clearly explained using the Factor theorem. If “k” is a zero of a polynomial P(x), then (x-k) is a factor of a given polynomial. The relation between the zeros and the coefficients of a polynomial is given below
Linear Polynomial
The linear polynomial is an expression, in which the degree of the polynomial is 1. The linear polynomial should be in the form of ax+b. Here, “x” is a variable, “a” and “b” are constant.
The polynomial P(x) is ax+b, then the zero of a polynomial is -b/a = – constant term/coefficient of x)
Quadratic Polynomial
The Quadratic polynomial is defined as a polynomial with the highest degree of 2. The quadratic polynomial should be in the form of ax2 + bx + c. In this case, a ≠ 0. Let say α and β are the two zeros of a polynomial, then
The sum of zeros, α + β is -b/a = – Coefficient of x/ Coefficient of x2
The product of zeros, αβ is c/a = Constant term / Coefficient of x2
Cubic Polynomial
The cubic polynomial is a polynomial with the highest degree of 3. The cubic polynomial should be in the form of ax3 + bx2 + cx + d, where a ≠ 0. Let say α, β, and γ are the three zeros of a polynomial, then
The sum of zeros, α + β + γ is -b/a = – Coefficient of x2/ coefficient of x3
The sum of the product of zeros, αβ+ βγ + αγ is c/a = Coefficient of x/Coefficient of x3
The product of zeros, αβγ is -d/a = – Constant term/Coefficient of x3
Zeros of a Polynomial Solved Examples
Example Evaluate the sum and product of zeros of the quadratic polynomial 4x2 – 9.
Solution
Given quadratic polynomial is 4x2 – 9.
4x2 – 9 can be written as 2x2 – 33, which is equal to (2x+3)(2x-3).
To find the zeros of a polynomial, equate the above expression to 0
(2x+3)(2x-3) = 0
2x+3 = 0
2x = -3
X = -3/2
Similarly, 2x-3 = 0,
2x = 3
x = 3/2
Therefore, the zeros of a given quadratic polynomial is 3/2 and -3/2.
Finding the sum and product of a polynomial:
The sum of the zeros = (3/2)+ (-3/2) = (3/2)-(3/2) = 0
The product of zeros = (3/2).(-3/2) = -9/4.
Viète's Formulas
François Viète, a French mathematician from the 16th century, provided us with elegant formulas that express the relationship between the zeroes and coefficients of a polynomial. For a quadratic polynomial.
Practical Applications:
Understanding this relationship has practical implications. By knowing the sum and product of zeroes, one can deduce information about the coefficients, aiding in solving equations and analyzing the behavior of polynomials.
CBSE Class 10th Downloadable Resources:
| 1. CBSE Class 10th Topic Wise Summary | View Page / Download |
| 2. CBSE Class 10th NCERT Books | View Page / Download |
| 3. CBSE Class 10th NCERT Solutions | View Page / Download |
| 4. CBSE Class 10th Exemplar | View Page / Download |
| 5. CBSE Class 10th Previous Year Papers | View Page / Download |
| 6. CBSE Class 10th Sample Papers | View Page / Download |
| 7. CBSE Class 10th Question Bank | View Page / Download |
| 8. CBSE Class 10th Topic Wise Revision Notes | View Page / Download |
| 9. CBSE Class 10th Last Minutes Preparation Resources (LMP) | View Page / Download |
| 10. CBSE Class 10th Best Reference Books | View Page / Download |
| 11. CBSE Class 10th Formula Booklet | View Page / Download |
Being in CBSE class 10th and considering the board examinations you must be needing resources to excel in your examinations. At TestprepKart we take great pride in providing CBSE class 10th all study resources in downloadable form for you to keep you going.
Below is the list of all CBSE class 10th Downloads available on TestprepKart for both Indian and NRI students preparing for CBSE class 10th in UAE, Oman, Qatar, Kuwait & Bahrain.
SAMPLE PRACTICE QUESTION
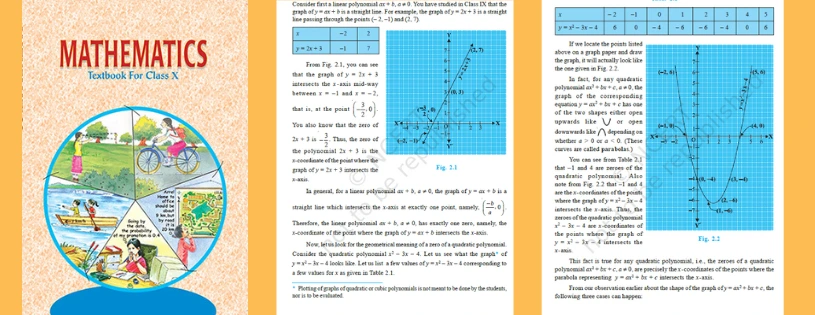
Q1: What is the geometrical meaning of the zeroes of a polynomial?
Ans: The zeroes of a polynomial represent the x-values at which the polynomial intersects the x-axis on a graph, indicating points where the polynomial evaluates to zero.
Q2: How do you visually interpret the zeroes on a graph?
Ans: Zeroes correspond to the x-coordinates where the polynomial function crosses or touches the x-axis, providing key information about the roots.
Q3: Can a polynomial have complex or imaginary zeroes with no real intersection on the graph?
Ans: Yes, a polynomial can have complex or imaginary zeroes that don't intersect the real axis on the graph, but they still contribute to the overall understanding of the polynomial's behavior.
Q4: How do multiple zeroes of a polynomial affect the shape of its graph?
Ans: The number of zeroes influences the graph's behavior. Double zeroes may create points of inflection, while triple zeroes may result in a flattened curve at the x-axis.
Q5: Do all polynomial graphs intersect the x-axis at the zeroes?
Ans: Not necessarily. The graph may touch the x-axis at a zero (with multiplicity one) or cross it (with odd multiplicity), influencing the graph's behavior around those points.

| CBSE CLASS 10 Mathematics Chapter |
| Chapter:1 Real Numbers |
| Chapter:2 Polynomials |
| > Introduction |
| > Geometrical Meaning of the Zeroes of a Polynomial |
| Chapter:4 Quadratic Equations |
| Chapter:5 Arithmetic Progressions |
| Chapter:6 Triangles |
| Chapter:7 Coordinate Geometry |
| Chapter:8 Introduction to Trigonometry |
| Chapter:9 Some Applications of Trigonometry |
| Chapter:10 Circles |
| Chapter:11 Areas Related to Circles |
| Chapter:12 Surface Areas and Volumes |
| Chapter:13 Statistics |
| Chapter:14 Probability |
| CBSE CLASS 10 Science Chapter |
| Chapter:1 Chemical Reactions and Equations |
| Chapter:2 Acids, Bases and Salts |
| Chapter:3 Metals and Non-metals |
| Chapter:4 Carbon and its Compounds |
| Chapter:5 Life Processes |
| Chapter:6 Control and Coordination |
| Chapter:7 How do Organisms Reproduce? |
| Chapter:8 Heredity |
| Chapter:9 Light – Reflection and Refraction |
| Chapter:10 The Human Eye and the Colourful World |
| Chapter:11 Electricity |
| Chapter:12 Magnetic Effects of Electric Current |
| Chapter:13 Our Environment |
| Class 8 |
| Class 9 |
| Class 11 |
| Class 12 |