Rational Numbers can be defined as numbers that can be written in the form of p/q where q≠0. Any fraction having zero as a denominator is not considered as a rational number. For example – 1/2, 2/3, 8/9, 0/3, and 0/7 are rational numbers but 4/0,7/0,8/0 are not rational, because they give the infinite value on dividing by zero.

In this article, we learn about rational numbers, properties of rational numbers, types of rational numbers, and the difference between rational numbers.

Get Maths Help Download DASA/CIWG E-BOOK

What are Rational Numbers 

In mathematics, Rational Numbers are defined as in the form of p/q where q≠0, or we can also say that fractions that fit in the rational numbers where numerator can be zero but Denominator not equal to zero in the when we divide the rational numbers we get a decimal form, which may be repeating decimal.

Book a Free Mathematics Class

How to identify Rational Numbers 

Identification of rational numbers can be checked by:-

• It is in the form of p/q where q≠0.

• The ration p/q can be solved in decimal form.

• Rational numbers can be positive, negative, and zero 

• They are non-terminating repeating decimals. 

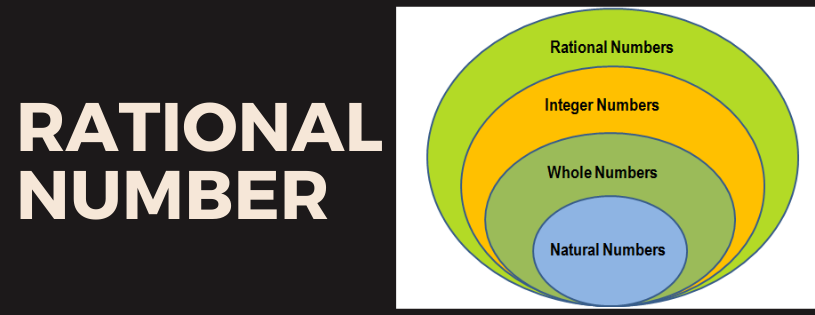
Types of rational numbers

There are different types of rational numbers are as given below:-

• They can be integers like -7,3,0

• They should be terminating decimals like 0.76,0.42.0.48.

•  They can be Non terminating decimals like 0.333,0.141414.

• They should be in the form of negative fractions -3/9,-9/5.

Book a Free Mathematics Class

Standard of Rational Numbers

The standard form of rational numbers can be defined as there is no common factor between dividend and divisor and hence divisor is positive.

For example, 13/26 is a rational number, but it can also be expressed in 1/2 common factor between dividend and divisor is only one, hence we can say 1/3  is in standard form.

Positive and Negative Rational Numbers 

We have known that a rational number can be written in the form of p/q where q≠0, where p and q are the integers. The rational number can be positive or negative. If p and q are positive integers then the rational number must be positive (p/q) while if integers p and q are negative, then rational numbers must be negative -(p/q).

Arithmetic Operations in Rational Numbers 

In arithmetic operations, we generally do addition, subtraction, multiplying, and dividing, now we see how can a rational number can be added, subtracted, multiplied, and divided.

In addition- when we add p/q and r/s, we need the same denominator for both fractions.

Example- (5/3) + (4/3) = (5+4/3) = 9/3.

Subtraction- Like addition, the subtraction can also be done with a rational number with the denominator being the same.

Example- (7/3) – (5/3) = (7-5/3) = 2/3  

Multiplication- In multiplication, the numerator and denominator are multiplied respectively. For example, s/t and u/v then on multiplication (s x u) and (t x v).

Example- 1/6  x 2/7 = 2/42.

Division- In Divison if (p/q) ፥ (r/s) then we get (ps) /(qr).

Example- (1/4) ፥ (1/3) = (3/4)

Rational and Irrational Numbers 

Rational numbers can be defined as positive, negative, and zero, and when we specify a negative rational number, the negative sign is either in the numerator or in front of the fraction of numbers.

Irrational numbers cannot be written as simple fractions they are represented with a decimal, and they have endless non-repeating numbers after the decimal point, examples of irrational numbers are –

Pi (π ) = 3.142857

2 =1.414213…

Solved Examples

Que 1- Identify the Mix fraction 1 ¹⁄₂, is that a rational number?

Ans- we simplify this equation = 3/2 

              Here numerator is 3

               And denominator is 2

          Hence, 3/2 is a rational number

Que 2- identify the following numbers rational or irrational  2/3, 100/2300, 15, 5.

Ans- 2/3  in the fraction with denominator ≠ 0 hence 2/3  is a rational number.

        100/2300 is a rational number.

         15 can be written as a 15/1 hence 15 is a rational number.

            5 = 2.2360679775….  is a non-terminating value hence it cannot be written infractions.

Hence it is an irrational number.

Que 3- Find a rational number between 1/2  and 2/3. 

Ans- We find the rational number between 1/2  and 2/3 by finding the average of both.

 Hence = 1/2  + 3/2       

     2

      = on solving this equation, we get

       = 7/12

Que 4- Multiply these rational number (2/5) x (5/2)

Ans-  (2/5) x (5/2)=  (2×5)/(5x 2) = 10/10 =1

Que 5- Divide the rational no (1/3) ፥ (1/2)

 Ans-  on dividing these two we get 

  =  (1/3) ፥ (1/2)

  = (3/2)